Revisiting basic statistics
A friend of mine works for a physical therapy clinic and she needed to decide if a certain patient's physical ability -- in this case, his hand grip strength -- is good enough. She let the patient use a strength measuring device and did 3 trial measurements. The device comes with a table of typical strengths (giving the average strength, μ, and the standard deviation, σ) for different age groups and gender.
From the 3 measurements, she calculated the average measured strength of the patient's hand grip. My friend's problem starts because the average measured strength is lower than the patient's age group's lower limit, μ-σ. Her question is: how can she trust the average of her 3 measurements, and thus conclude safely that the patient really has a weak hand grip?
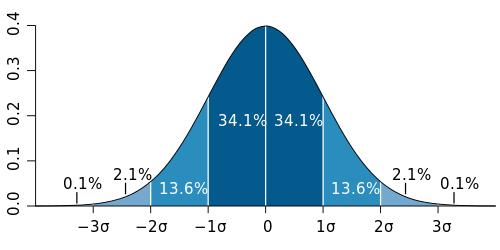
To answer this question, we have to make an assumption that if the patient would have to make a large number (n) of trials, the measured grip strengths would vary or fluctuate around the average measurement and that if we draw a graph of the distribution of measurements, we would see a "bell curve", as shown below.
This curve is also known as the normal distribution, and is often the behavior of the result of measurement of many natural quantities.
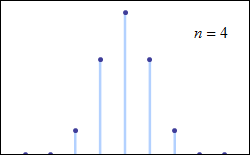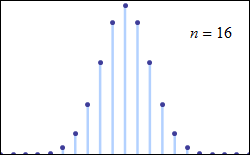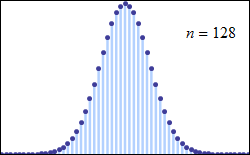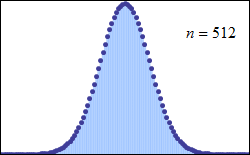
But in most practical uses, we won't have data that will look like this smooth bell curve. Instead, what we have is a discrete graph of measured data that can only approach the bell curve when the number (n) of sample measurements becomes large enough, similar to this graph below:
As a consequence, we can see that the calculation of the average measurement can only be very reliable when the number (n) of sample measurements is large enough. But my friend will have to live with just 3 trial measurements (and the poor patient probably can't do a lot of tests). So is there a way she can test the usability of her results? Luckily, there is.
The average measured grip strength has a precision, ε, that can be calculated based on the standard deviation σ of the data and of number (n) of trials: ε = σ / sqrt( n ). Then we relate the value of ε to the coefficient of variation, σ/μ, of her measured data, which she calculated to be 0.15: ε/μ = (σ/μ)(1/sqrt( n)).
So, for just 3 trials, ε/μ = 0.15 / sqrt( 3 ) = 0.087. That is, the precision of the average measured grip strength is about 8.7% of its value. Hence, we can say that her measurements were precise enough and that the poor patient's grip strength is really lower than μ-σ. Simply said, the poor guy's hand grip is really weaker than more than 84% of his age group.
Disclaimer: Of course, my friend will have to decide for herself the proper course of treatment for her patient and this discussion should be taken with a grain of salt. For one, I have a feeling that the actual distribution for the higher-age groups don't really follow the normal distribution.
Related reading(s):






From the 3 measurements, she calculated the average measured strength of the patient's hand grip. My friend's problem starts because the average measured strength is lower than the patient's age group's lower limit, μ-σ. Her question is: how can she trust the average of her 3 measurements, and thus conclude safely that the patient really has a weak hand grip?
To answer this question, we have to make an assumption that if the patient would have to make a large number (n) of trials, the measured grip strengths would vary or fluctuate around the average measurement and that if we draw a graph of the distribution of measurements, we would see a "bell curve", as shown below.
But in most practical uses, we won't have data that will look like this smooth bell curve. Instead, what we have is a discrete graph of measured data that can only approach the bell curve when the number (n) of sample measurements becomes large enough, similar to this graph below:
As a consequence, we can see that the calculation of the average measurement can only be very reliable when the number (n) of sample measurements is large enough. But my friend will have to live with just 3 trial measurements (and the poor patient probably can't do a lot of tests). So is there a way she can test the usability of her results? Luckily, there is.
The average measured grip strength has a precision, ε, that can be calculated based on the standard deviation σ of the data and of number (n) of trials: ε = σ / sqrt( n ). Then we relate the value of ε to the coefficient of variation, σ/μ, of her measured data, which she calculated to be 0.15: ε/μ = (σ/μ)(1/sqrt( n)).
So, for just 3 trials, ε/μ = 0.15 / sqrt( 3 ) = 0.087. That is, the precision of the average measured grip strength is about 8.7% of its value. Hence, we can say that her measurements were precise enough and that the poor patient's grip strength is really lower than μ-σ. Simply said, the poor guy's hand grip is really weaker than more than 84% of his age group.
Disclaimer: Of course, my friend will have to decide for herself the proper course of treatment for her patient and this discussion should be taken with a grain of salt. For one, I have a feeling that the actual distribution for the higher-age groups don't really follow the normal distribution.
Related reading(s):



Comments